Implications of photon-ALP oscillations in the extragalactic neutrino source TXS 0506+056 at sub-PeV energies
Abstract
Photon-axion-like particle (ALP) oscillations result in the survival of gamma rays from distant sources above TeV energies. Studies of events observed by CAST, Fermi-LAT, and IACT have constrained the ALP parameters. We investigate the effect of photon-ALP oscillations on the gamma-ray spectra of the first extragalactic neutrino source, TXS 0506+056, for observations by Fermi-LAT and MAGIC around the IC170922-A alert. We obtain a constraint on the ALP coupling parameter GeV-1 with 95% C.L. when focusing on the ALP mass range 0.1 neV 1000 neV. Importantly, we study the implications of ALP- oscillations on the counterpart rays of the sub-PeV neutrinos observed from TXS 0506+056. We also show the diffuse -ray fluxes and observabilities from flat-spectrum radio quasars, high-synchrotron peaked sources, and low-intermediate-synchrotron peaked sources, assuming similar gamma-ray emissions as that from TXS 0506+056.
I Introduction
Axion-like particles (ALPs) are pseudoscalar (spin-0) bosons with very light mass and are potential candidates for dark matter [1, 2]. The axions are also proposed to solve the problem in QCD [3, 4]. In the presence of an external magnetic field, ALPs can couple to photons via two coupling vertices which leads to the photon-ALP oscillation. In astrophysical environments, photon-ALP conversion drastically reduces the absorption of very-high-energy (VHE) photons by extragalactic background light (EBL) and the cosmic microwave background (CMB) through pair production above 100 GeV [5, 6, 7, 8, 9, 10].
This increased transparency can modulate and enhance the observed -ray spectra of the TeV photons originating from higher-redshift sources using observations of -ray spectra from VHE sources [11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25, 26, 27, 28] to set stringent constraints on the ALP mass, , and coupling constant . Interestingly, the recent observations of nearly 18-TeV photons by the Large High Altitude Air Shower Observatory (LHAASO) with the kilometer-square area (KM2A) [29] and an astonishing 251-TeV photon by Carpet-2 [30] from the long gamma-ray burst GRB 221009A at redshift 0.1505 has motivated the community to understand the survival of photons at this energy through ALP-photon oscillation [31, 32]. We note that the above-mentioned 251-TeV photon observed by Carpet-2 also has the candidate sources LHAASO J1929+1745 and 3HWC J1928+178, as reported in Ref. [33]. Most of these studies focused on photons at energies observed by the Imaging Atmospheric (or Air) Cherenkov Telescope (IACT). Observations from the axion flux of the Sun have also been studied by the CERN Axion Solar Telescope (CAST), giving the most stringent constraint on the ALP parameters, eV, 6.610-11 GeV-1 [34, 35].
The effect of ALP-photon oscillation at sub-PeV energies and higher has recently been explored with Galactic diffuse gamma rays using High Altitude Water Cherenkov (HAWC), Tibet AS-, and LHAASO events, resulting in a limit of eV, GeV-1 with 95% confidence limit (C.L.) [36], and excluding GeV-1 for eV [37] at 95% C.L., respectively. The intrinsic photon flux would be different at these energies than at lower energies due to the addition of hadronic channels. With the observations of sub-PeV neutrinos, we may understand the energetics of the source.
In this work, we investigate the implications of photon-ALP oscillation for the first ever non-Galactic sub-PeV neutrino source [38] TXS 0506+056 situated at a redshift [39]. It was first discovered as a radio source [40] and later as high-energy gamma radiation with space missions, like the Energetic Gamma Ray Experiment Telescope and Fermi-Large Area Telescope (Fermi-LAT)[41, 42, 43]. On September 22, 2017 (IC170922-A), the IceCube Neutrino Observatory detected a very-high-energy 290-TeV muon neutrino coinciding with the direction of a flaring state of TXS 0506+056 [44]. Soon, follow-up observations were performed in various energy bands by Fermi-LAT ( rays) [45], the Nuclear Spectroscopic Telescope Array (X rays) [46], and Swift (X rays, UV, optical) [47], and VHE -ray observations were made by the Major Atmospheric Gamma Imaging Cherenkov Telescopes (MAGIC) [48], High Energy Stereoscopic System [49], HAWC [50] and Very Energetic Radiation Imaging Telescope Array System [51]. Notably, prior to the IC170922-A alert, this source was also observed with a neutrino flare, making it a sub-PeV neutrino source with a significance of 3.5 [52]. However, there was no significant flaring in MeV-GeV gamma rays during this epoch. A hadronic-originated photon counterpart will contribute to the intrinsic flux at sub-PeV for such neutrino sources. Hence, TXS 0506+056 is the candidate source to study the ALP-photon oscillation at several-TeV to sub-PeV energies.
This paper is organized as follows. In Sec. II, we describe the propagation of photon-ALP beam in an external magnetic field. In Sec. III we describe the various magnetic field models used for the analysis. In Sec. IV we discuss the methodology used for data fitting and predicting the expected -ray flux. In Sec. V we describe the significance of the ALP effect in TXS 0506+056 and its Fermi-LAT analysis. In Sec. VI we discuss the results for the ALP- oscillations. This section also includes the implication of this oscillation for the diffuse gamma-ray flux from TXS 0506+056-like sources. Here we calculate the diffuse gamma-ray flux from sources, flat-spectrum radio quasars(FSRQs), high-synchrotron peaked (HSP) sources, and low-intermediate-synchrotron peaked (LISP) sources, and their future observability.
II Photon-ALP Oscillation and Propagation in Magnetic Fields
The minimal interaction coupling between photons of energy and ALPs in the presence of an external magnetic field B and electric field E has been proposed in the literature [53, 5].
A polarized, monoenergetic photon beam propagating along the direction in a cold plasma medium with a homogeneous B field along the axis, has the equation of motion,
| (1) |
where represents the photon-ALP mixing matrix and denotes the state function. Here, and are the photon amplitudes with linear polarizations along the x and y axis, respectively, whereas is the amplitude associated with the ALP state.
Assuming weak magnetic fields and at VHE, the QED vacuum polarization and Faraday rotation can be neglected, and the mixing matrix becomes
| (2) |
where , , and . Here, is the plasma frequency resulting from the charge-screening effect. The propagation region of the photon-ALP beam is divided into N subregions. In each region, the probability for photon survival is calculated. The initial beam state is
| (3) |
The final photon survival probability can be written as:
| (4) |
where diag(1, 0, 0) and diag(0, 1, 0) denote the polarization along the x and y axis, respectively, and is the whole propagation transfer matrix. Here, the subregions are the source, the extragalactic medium, and the Milky Way region.
III Magnetic field models
In this section, we give a brief overview of the magnetic field models used in our calculation.
III.1 Blazar jet region
We consider the photon-ALP oscillation at the source in the presence of blazar jet magnetic field (BJMF). The magnetic field in the jet region can be modeled with poloidal (along the jet axis, ) and toroidal (transverse to the jet axis, ) components. At distances large enough from the central black hole, the toroidal component dominates over the poloidal component and thus the latter can be neglected. We adopt the toroidal magnetic field strength given by [54, 55]:
| (5) |
where is the magnetic field strength at the core and is the distance between the VHE -ray-emitting region and the central black hole. We assume , where is the blob radius of the VHE emitting region and is the angle between the jet axis and the line of sight.
Assuming equipartition between the magnetic field and particle energies, the electron density profile can be modeled as a power law given by [56]:
| (6) |
where is the electron density at . In Ref. [57], a more realistic model was provided that takes into account the fact that the electron distribution is nonthermal in a relativistic active galactic nuclei Jet.
III.2 Intercluster magnetic fields
The intercluster medium magnetic field (ICMF), can be modeled as,
| (7) |
with , where and are the magnetic field strength and electron density at the cluster center, respectively. The electron density distribution at a distance from the cluster center is
| (8) |
with is the core radius and . The typical order of the electron density and the core radius is cm-3 and kpc, respectively.
In a cluster-rich environment, the turbulent magnetic field is of order (1)G [58, 59, 60]. Such a cluster can have a significant effect on the conversion between photons and ALPs [61, 62]. Since there is no evidence that TXS 0506+056 is located in a cluster-rich environment, we do not consider the photon-ALP oscillations in the ICMF model.
III.3 Extragalactic magnetic fields
The actual strength of the extragalactic magnetic field on the cosmological scale (1) Mpc, is still unknown, but the currently accepted limit is (1) nG [63, 64]. In this work, we neglect the effect due to the magnetic field (See Ref. [65] for possible effects of the magnetic field) and consider only the absorption effect due to EBL.
The optical depth of EBL attenuation can be written as [66]
| (9) |
with
| (10) |
and where is the redshift of the source, is the rate of Hubble expansion, is the threshold energy for pair production, is the proper number density of the EBL, is the pair-production cross section, is the angle between the projectile and target photons, is the projectile photon energy, and is the target background photon energy. Several EBL models have been proposed in the literature [67, 68, 69, 70, 71, 72, 73], and we consider the model from Ref. [74] in this work.
III.4 Milky Way region
Finally, we consider the effect of photon-ALP oscillation in the presence of a Galactic magnetic field (GMF). This effect can have both a large-scale regular component and a small-scale random component. Due to the fact that the coherence length is smaller than the oscillation length, we neglect the random component [61] in our analysis and consider only the regular GMF component model given in [75]; the latest model can be found in Refs. [76, 77].
IV Methodology
The photon beam of the blazar can be considered as the intrinsic photons generated by the accelerated leptons or hadrons. In general, one can consider the intrinsic spectrum to follow the superexponential cutoff power law (SEPWL) to fit the observed data points under the null hypothesis,
| (11) |
where is taken to be 1 GeV, and , , , and are treated as free parameters. The best-fit parameters are given in Table 1. It is to be noted that we also tested other forms of the intrinsic spectrum and found that the SEPWL gives the smallest value of the best-fit under the null hypothesis.
| Phase | (x10-11) | |||
|---|---|---|---|---|
| [MeV-1cm-2s-1] | [GeV] | |||
| Neutrino Flare 2014 | 0.65 | 1.79 | 0.1 | 71.01 |
| VHE Flare 1 | 4.04 | 1.99 | 0.54 | 66.27 |
| VHE Quiescent | 1.59 | 1.94 | 0.63 | 58.37 |
We use the open-source PYTHON-based package gammaALPs111https://gammaalps.readthedocs.io/en/latest/index.html [78] to compute the photon-ALP oscillation probability .
The modulated -ray spectrum obtained after the photon-ALP oscillation is given by . In order to get the expected -ray spectrum observed in the detector, we must consider the energy resolution of the detector. One can assume the energy dispersion function to be a Gaussian with the variance being the energy resolution. Then the expected flux between energy bins and is given by [26]
| (12) |
where is the true energy of rays. The energy resolution of Fermi-LAT and MAGIC are taken as 222https://fermi.gsfc.nasa.gov/ssc/data/analysis/documentation/Cicerone/Cicerone_Introduction/LAT_overview.html and [79], respectively.
One can obtain the best-fit photon-ALP oscillation probability by fitting the observed flux with the expected flux. We define as,
| (13) |
where is the observed -ray flux, with being the corresponding uncertainty. The best-fit and are calculated by minimizing the .
V Significance of ALP effect in TXS 0506+056
The first extragalactic TeV-PeV neutrino source TXS 0506+056 is the best candidate to study high-energy radiation. The follow-up observations of IC170922-A showed that the source has multiwavelength emissions. Additionally, the source showed an excess of in the time window of 158 days, from September 2014 (MJD 56937.81) to March 2015 (MJD 57096.21), known as the neutrino flare phase [52]. Recently, 41-hr and -hr observations of VHE ( GeV) events by MAGIC between September 2017 to December 2018 showed three flaring activities [80, 81]. Hence, the blazar TXS 0506+056 would be the best probe to study the photon-ALP interaction in the MeV to the sub-PeV range. We study the VHE activity phases as well as the neutrino-flare phase of the source.
V.1 Fermi-LAT analysis of TXS 0506+056
We analyze the Fermi-LAT data for TXS 0506+056 in three phases:
-
•
Neutrino Flare : September 2014 (MJD 56937.81) to March 2015 (MJD 57096.21).
-
•
VHE Flare 1 : 04 September, 2017 (MJD 58000) to 03 November, 2017 (MJD 58060).
-
•
VHE Quiescent : 04 November, 2017 (MJD 58061) to 10 October, 2018 (MJD 58422).
We select the above-mentioned time period of Fermi-LAT (Pass 8) processed data from the Fermi Science Data Center 333https://fermi.gsfc.nasa.gov/ssc/data/access/. We choose the spacecraft files and the instrument response functions (IRFs) of P8R3_SOURCE_V2 to match the extracted data. We select the SOURCE event class (evclass=128 and evtype=3) in the energy range of 100 MeV to 300 GeV. The region of interest is selected to be 2∘ centered on the target source. We consider all of the 4FGL sources around 10∘ of TXS 0506+056 as background sources together with preprocessed templates of Galactic diffuse emission, gll_iem_v08.fits , and the extragalactic isotropic diffuse emission, iso_P8R3_SOURCE_V2_v2.fits.
The likelihood analysis, spectral energy distribution, and light curve are obtained by using the PYTHON-based Fermipy package 444https://fermipy.readthedocs.io/en/latest/index.html [82].
VI Results and Discussions
We divide this section into two important parts: results obtained by calculating the oscillation probability, and discussions on the possible gamma rays at sub-PeV energies resulting from this oscillation.
VI.1 Constraints on ALP parameter space
We calculate the photon-ALP oscillation probability for the three phases of TXS0506+056 mentioned above following Sec. IV.
VI.1.1 VHE activity around IC170922-A
In this section, we discuss the results obtained for VHE Flare 1, and VHE Quiescent associated with the IC170922-A event using the Fermi-LAT and MAGIC events. The VHE events observed by MAGIC are collected from Ref. [80, 81]. We use the modeling parameters for these phases from [81]. The parameters used in the BJMF model in the gammaALPs package are listed in Table 2. Note that for this study we do not consider the ICMF model.
| Parameter name | Quiescent | Flaring |
| R.A.(J2000) | 05 09 25 (hh mm ss) | ” |
| Dec.(J2000) | +05 42 09 (dd mm ss) | ” |
| z | 0.337 | ” |
| [deg] | 0.8 | ” |
| 40 | ” | |
| 22 | ” | |
| [G] | 1 | ” |
| [cm-3] | 520 | |
| [pc] | 10 | ” |
| [ cm] | 1.1 | ” |
| -1 | ” | |
| -2 | ” | |
| 800 | ” | |
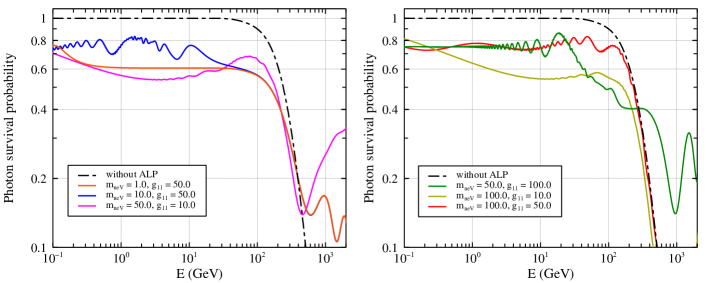
Figure 1 shows the photon survival probability due to EBL/CMB and photon-ALP oscillations for several typical sets of and .
Figure 2 shows the distribution of in the parameter space for all three VHE activity phases. In Table 3, we summarize the best-fit and values along with the parameters obtained under the null and ALP hypotheses. To summarize, we show the -ray flux for the best-fit values along with the final photon survival probability in Fig. 4.
| Phase | |||||
|---|---|---|---|---|---|
| Neutrino Flare 2014 | 5.65 | 3.31 | 4.47 | 39.81 | 6.72 |
| VHE Flare 1 | 20.48 | 13.73 | 17.78 | 35.48 | 9.18 |
| VHE Quiescent | 28.79 | 24.47 | 11.22 | 94.41 | 11.81 |
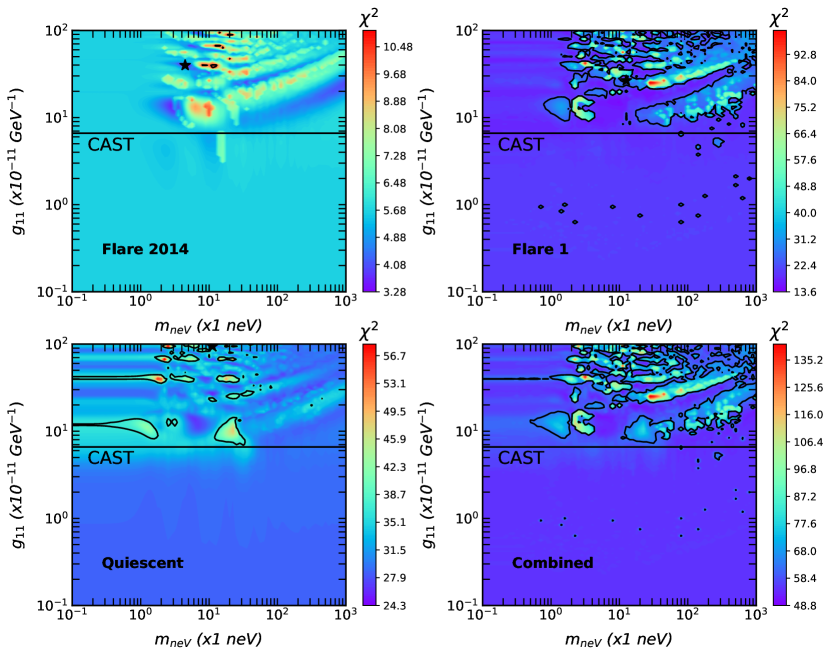
To set the constraint on the – parameter space, a threshold value is determined to exclude the region at a certain C.L. for each phase. We generate 400 sets of pseudodata realized by Gaussian samplings as in Ref. [22], with the mean value and standard deviation taken as the best-fit flux under the null hypothesis and errors on the experimental data, respectively.
For each set, we calculate the best-fit for both the null and ALP hypotheses using the method described in Sec. IV. We obtain the distribution of test statistics (TS) values, under the null hypothesis that obeys the noncentral distribution. The assumption that the probability distribution for the ALP scenario can be approximated with the null hypothesis, , is derived at 95 C.L. as shown in Fig. 3. The black contours in Fig. 2 represent the excluded parameter space at 95 C.L. We find the best constraint on – parameter space in the Flare 1 phase.
In this phase, we find a possible excluding constraint on that can go as low as GeV-1 within 95% C.L.. TXS 0506+056 is a variable blazar with a variability index of 245.9099, and significant determination of the intrinsic flux is difficult for a flaring blazar. Thus we obtain weaker constraints for ALP parameters. We even see this result in the combined analysis of the three phases. On the other hand, for the Quiescent phase, this value could reach the similar constraint to that found by the CAST experiment.
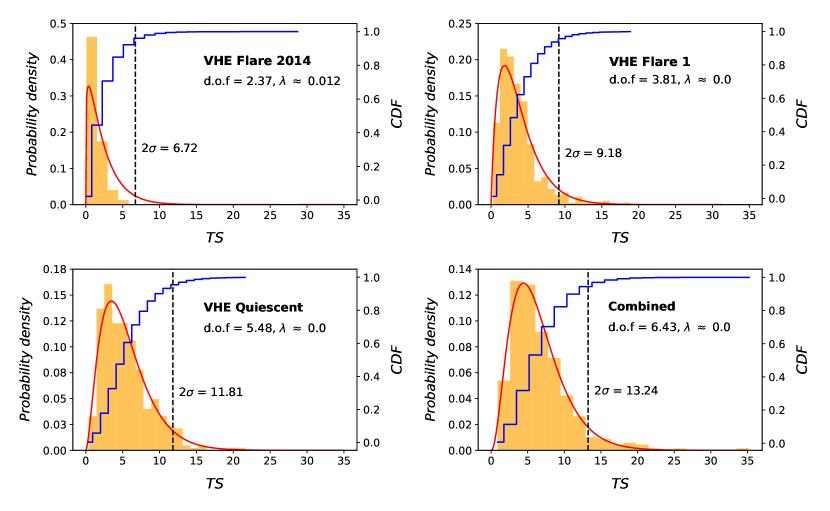
VI.1.2 Neutrino flare (2014-2015)
We also investigate the ALP effect for the neutrino flare phase of the blazar TXS 0506+056. For the neutrino flare, there were no VHE observations. Hence we calculated the best-fit oscillation probability using only the Fermi-LAT events following the method as in Sec. IV. However, this phase could not add any significant result to the – parameter space.
VI.2 ALP effect at sub-PeV energies
Extending this survival probability to sub-PeV energies, we can estimate the residual photon flux of the observed neutrino’s counterpart. For this calculation, we use the ALP- oscillation using the CAST upper limits on the parameters, namely neV and GeV-1.
Assuming that IC170922-A resulted from proton-proton interactions, as calculated in Ref. [83], the average counterpart rays will follow [84], where is the energy of the photons produced from decay. Subsequently, these VHE photons attenuate by interacting with the synchrotron and synchrotron self-Compton photons, resulting from relativistic electrons inside the blob. The fraction of VHE photons that can escape from the blob is estimated as in Ref. [85],
| (14) |
where the optical depth due to the interaction of high-energy photons of energy in the comoving frame is
| (15) |
is the number density of the ambient photons of energy (in ) in the comoving frame,
| (16) |
and is the pair-production cross section [86]. is the radius of the blob, and is the luminosity distance of the source. is the bulk Lorentz factor and is the photon flux in the observer frame.
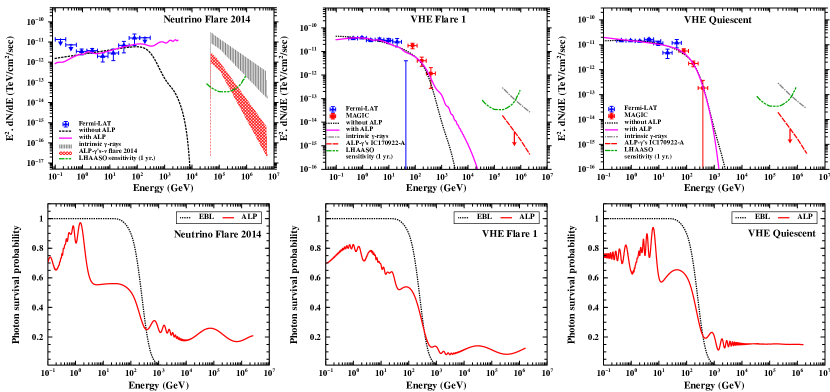
Hence, the intrinsic -ray spectrum can be obtained by counterpart gamma rays as in Sec. VI.2 and this is shown by the grey vertical hatched and dot-dashed curves in the top panel of Fig. 4. This flux generally gets exhausted due to interaction with the EBL and CMB. On multiplying the intrinsic flux with the photon survival probability under photon-ALP oscillations for the corresponding phase, we can have a surviving fraction of the flux. The rays due to the ALP effect at sub-PeV energies is shown by the red cross hatched and dashed curves. Interestingly, the surviving photons, considering the ALP- oscillations in VHE Flare 2014, are above the differential sensitivity to a Crab-like point gamma-ray source for 1 year of exposure by LHAASO [87]..
VI.3 Diffuse rays from FSRQs sources at sub-PeV energies due to ALP effect
In this section, we calculate the diffuse flux at sub-PeV energies as an effect of ALP- oscillation in sources like TXS 0506+056. The authors of Refs. [88, 38] emphasized that TXS 0506+056 cannot be considered a blazar of BL Lac type, but rather an intrinsically FSRQ, and all FSRQs are of the low-energy (synchrotron) peaked (LBL). Hence, we calculate the diffuse ALP flux for the source luminosity function (LF) evolution like FSRQs using
| (17) | |||||
where is the intrinsic photon index distribution which is assumed to be a Gaussian, is the comoving volume element per unit redshift per unit solid angle, is the intrinsic photon flux, here taken as calculated in Sec. VI.2 for TXS 0506+056, is the opacity under the ALP scenario considering the CAST upper limit on the parameters – , and is the gamma-ray luminosity function (GLF).
We consider here the luminosity-dependent density evolution of the GLF with parametrization given by:
| (18) | |||||
with
| (19) |
where . We collect the best-fit parameters , and for three blazar classes, namely, FSRQ [89], HSP, and LISP sources [90]. The limits of integration are , , , and . For FSRQs, we also calculate the diffuse flux using the integration limits of Ref. [89] as a comparison.
Figure 5 shows the diffuse ray flux expected from photon-ALP conversion for the three classes. The data points of diffuse gamma-ray emission from the Galactic plane recorded by the LHAASO-KM2A [91] and Tibet AS- [92] experiments are also shown. We show each class with (i) erg/sec, and (ii) erg/sec.
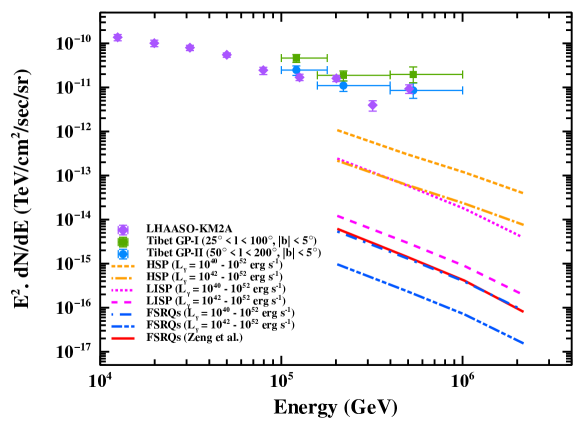
Dedicated surveys of the extragalactic diffuse gamma-ray flux by observatories like LHAASO, Tibet AS-, and the upcoming Cerenkov Telescope Array [93] will be able to constrain the ALP parameters considering photon-ALP oscillations at sub-PeV energies as proposed here.
Acknowledgements.
The authors thank the anonymous referee for constructive comments which helped in improving the manuscript. The authors acknowledge the Science and Engineering Research Board (SERB) Grant No. SRG/2020/001932.References
- Preskill et al. [1983] J. Preskill, M. B. Wise, and F. Wilczek, Physics Letters B 120, 127 (1983).
- Khlopov et al. [1999] M. Khlopov, A. Sakharov, and D. Sokoloff, Nuclear Physics B - Proceedings Supplements 72, 105 (1999), proceedings of the 5th IFT Workshop on Axions.
- Peccei and Quinn [1977] R. D. Peccei and H. R. Quinn, Phys. Rev. D 16, 1791 (1977).
- Sikivie [2010] P. Sikivie, International Journal of Modern Physics A 25, 554 (2010), https://doi.org/10.1142/S0217751X10048846 .
- De Angelis et al. [2011] A. De Angelis, G. Galanti, and M. Roncadelli, Phys. Rev. D 84, 105030 (2011).
- Reesman and Walker [2014] R. Reesman and T. Walker, Journal of Cosmology and Astroparticle Physics 2014 (08), 021.
- Tavecchio et al. [2015] F. Tavecchio, M. Roncadelli, and G. Galanti, Physics Letters B 744, 375 (2015).
- Galanti et al. [2019] G. Galanti, F. Tavecchio, M. Roncadelli, and C. Evoli, Monthly Notices of the Royal Astronomical Society 487, 123 (2019), https://academic.oup.com/mnras/article-pdf/487/1/123/28693814/stz1144.pdf .
- Li et al. [2022] H.-J. Li, X.-J. Bi, and P.-F. Yin, Chinese Physics C 46, 085105 (2022).
- Li [2022] H.-J. Li, Physics Letters B 829, 137047 (2022).
- Hooper and Serpico [2007] D. Hooper and P. D. Serpico, Phys. Rev. Lett. 99, 231102 (2007).
- Simet et al. [2008] M. Simet, D. Hooper, and P. D. Serpico, Phys. Rev. D 77, 063001 (2008).
- Tavecchio et al. [2012] F. Tavecchio, M. Roncadelli, G. Galanti, and G. Bonnoli, Phys. Rev. D 86, 085036 (2012).
- Meyer et al. [2013] M. Meyer, D. Horns, and M. Raue, Phys. Rev. D 87, 035027 (2013).
- Abramowski et al. [2013] A. Abramowski et al. (H.E.S.S. Collaboration), Phys. Rev. D 88, 102003 (2013).
- Ajello et al. [2016] M. Ajello et al. (The Fermi-LAT Collaboration), Phys. Rev. Lett. 116, 161101 (2016).
- Berenji et al. [2016] B. Berenji, J. Gaskins, and M. Meyer, Phys. Rev. D 93, 045019 (2016).
- Kohri and Kodama [2017] K. Kohri and H. Kodama, Phys. Rev. D 96, 051701 (2017).
- Meyer et al. [2017] M. Meyer, M. Giannotti, A. Mirizzi, J. Conrad, and M. A. Sánchez-Conde, Phys. Rev. Lett. 118, 011103 (2017).
- Galanti and Roncadelli [2018a] G. Galanti and M. Roncadelli, Journal of High Energy Astrophysics 20, 1 (2018a).
- Zhang et al. [2018] C. Zhang, Y.-F. Liang, S. Li, N.-H. Liao, L. Feng, Q. Yuan, Y.-Z. Fan, and Z.-Z. Ren, Phys. Rev. D 97, 063009 (2018).
- Liang et al. [2019] Y.-F. Liang, C. Zhang, Z.-Q. Xia, L. Feng, Q. Yuan, and Y.-Z. Fan, Journal of Cosmology and Astroparticle Physics 2019 (06), 042.
- Long et al. [2020] G. B. Long, W. P. Lin, P. H. T. Tam, and W. S. Zhu, Phys. Rev. D 101, 063004 (2020).
- Libanov and Troitsky [2020] M. Libanov and S. Troitsky, Physics Letters B 802, 135252 (2020).
- Bi et al. [2021] X. Bi, Y. Gao, J. Guo, N. Houston, T. Li, F. Xu, and X. Zhang, Phys. Rev. D 103, 043018 (2021).
- Guo et al. [2021] J.-G. Guo, H.-J. Li, X.-J. Bi, S.-J. Lin, and P.-F. Yin, Chinese Physics C 45, 025105 (2021).
- Gautham et al. [2021] A. Gautham, F. Calore, P. Carenza, M. Giannotti, D. Horns, J. Kuhlmann, J. Majumdar, A. Mirizzi, A. Ringwald, A. Sokolov, F. Stief, and Q. Yu, Journal of Cosmology and Astroparticle Physics 2021 (11), 036.
- Li et al. [2021] H.-J. Li, J.-G. Guo, X.-J. Bi, S.-J. Lin, and P.-F. Yin, Phys. Rev. D 103, 083003 (2021).
- Huang et al. [2022] Y. Huang et al. (LHAASO Collaboration), GRB Coordinates Network 32677, 1 (2022).
- Dzhappuev et al. [2022] D. D. Dzhappuev et al., The Astronomer’s Telegram 15669, 1 (2022).
- Galanti et al. [2022] G. Galanti, M. Roncadelli, and F. Tavecchio, arXiv e-prints , arXiv:2210.05659 (2022), arXiv:2210.05659 [astro-ph.HE] .
- Baktash et al. [2022] A. Baktash, D. Horns, and M. Meyer, arXiv e-prints , arXiv:2210.07172 (2022), arXiv:2210.07172 [astro-ph.HE] .
- Fraija et al. [2022] N. Fraija, M. Gonzalez, and HAWC Collaboration, The Astronomer’s Telegram 15675, 1 (2022).
- Zioutas et al. [1999] K. Zioutas et al., Nuclear Instruments and Methods in Physics Research Section A: Accelerators, Spectrometers, Detectors and Associated Equipment 425, 480 (1999).
- Anastassopoulos et al. [2017] V. Anastassopoulos et al., Nature Physics 13, 584 (2017), arXiv:1705.02290 [hep-ex] .
- Eckner and Calore [2022] C. Eckner and F. Calore, Phys. Rev. D 106, 083020 (2022).
- Mastrototaro et al. [2022] L. Mastrototaro, P. Carenza, M. Chianese, D. F. G. Fiorillo, G. Miele, A. Mirizzi, and D. Montanino, European Physical Journal C 82, 1012 (2022), arXiv:2206.08945 [hep-ph] .
- Padovani et al. [2018] P. Padovani, P. Giommi, E. Resconi, T. Glauch, B. Arsioli, N. Sahakyan, and M. Huber, Monthly Notices of the Royal Astronomical Society 480, 192 (2018), https://academic.oup.com/mnras/article-pdf/480/1/192/25231121/sty1852.pdf .
- Paiano et al. [2018] S. Paiano, R. Falomo, A. Treves, and R. Scarpa, The Astrophysical Journal Letters 854, L32 (2018).
- Lawrence et al. [1983] C. R. Lawrence, C. L. Bennett, J. A. Garcia-Barreto, P. E. Greenfield, and B. F. Burke, ApJS 51, 67 (1983).
- Halpern et al. [2003] J. P. Halpern, M. Eracleous, and J. R. Mattox, The Astronomical Journal 125, 572 (2003).
- Lamb and Macomb [1997] R. C. Lamb and D. J. Macomb, The Astrophysical Journal 488, 872 (1997).
- Abdo et al. [2010] A. A. Abdo et al., The Astrophysical Journal 715, 429 (2010).
- Aartsen et al. [2018] M. G. Aartsen et al., Science 361, eaat1378 (2018), arXiv:1807.08816 [astro-ph.HE] .
- Tanaka et al. [2017] Y. Tanaka, S. Buson, and D. Kocevski, Astronomer’s telegram (2017).
- Fox et al. [2017] D. B. Fox, J. J. DeLaunay, A. Keivani, P. A. Evans, C. F. Turley, J. A. Kennea, D. F. Cowen, J. P. Osborne, M. Santander, and F. E. Marshall, The Astronomer’s Telegram 10845, 1 (2017).
- Evans et al. [2017] P. A. Evans, A. Keivani, J. A. Kennea, D. B. Fox, D. F. Cowen, J. P. Osborne, F. E. Marshall, and Swift-IceCube Collaboration, The Astronomer’s Telegram 10792, 1 (2017).
- Mirzoyan [2017] R. Mirzoyan, The Astronomer’s Telegram 10817, 1 (2017).
- de Naurois et al. [2017] M. de Naurois et al. (H.E.S.S. Collaboration), The Astronomer’s Telegram 10787, 1 (2017).
- Martinez et al. [2017] I. Martinez, I. Taboada, M. Hui, and R. Lauer, The Astronomer’s Telegram 10802, 1 (2017).
- Mukherjee [2017] R. Mukherjee, The Astronomer’s Telegram 10833, 1 (2017).
- Collaboration et al. [2018] I. Collaboration, M. Aartsen, M. Ackermann, J. Adams, J. A. Aguilar, M. Ahlers, M. Ahrens, I. Al Samarai, D. Altmann, K. Andeen, et al., Science 361, 147 (2018).
- Raffelt and Stodolsky [1988] G. Raffelt and L. Stodolsky, Phys. Rev. D 37, 1237 (1988).
- Begelman et al. [1984] M. C. Begelman, R. D. Blandford, and M. J. Rees, Rev. Mod. Phys. 56, 255 (1984).
- Ghisellini and Tavecchio [2009] G. Ghisellini and F. Tavecchio, Monthly Notices of the Royal Astronomical Society 397, 985 (2009), https://academic.oup.com/mnras/article-pdf/397/2/985/2943748/mnras0397-0985.pdf .
- O’Sullivan and Gabuzda [2009] S. P. O’Sullivan and D. C. Gabuzda, Monthly Notices of the Royal Astronomical Society 400, 26 (2009), https://academic.oup.com/mnras/article-pdf/400/1/26/18690573/mnras0400-0026.pdf .
- Davies et al. [2022] J. Davies, M. Meyer, and G. Cotter, Phys. Rev. D 105, 023017 (2022).
- Carilli and Taylor [2002] C. Carilli and G. Taylor, Annual Review of Astronomy and Astrophysics 40, 319 (2002).
- Govoni and Feretti [2004] F. Govoni and L. Feretti, International Journal of Modern Physics D 13, 1549 (2004).
- Subramanian et al. [2006] K. Subramanian, A. Shukurov, and N. E. L. Haugen, Monthly Notices of the Royal Astronomical Society 366, 1437 (2006), https://academic.oup.com/mnras/article-pdf/366/4/1437/3915441/366-4-1437.pdf .
- Meyer et al. [2014] M. Meyer, D. Montanino, and J. Conrad, Journal of Cosmology and Astroparticle Physics 2014 (09), 003.
- Wouters and Brun [2012] D. Wouters and P. Brun, Phys. Rev. D 86, 043005 (2012).
- Ade et al. [2016] P. A. R. Ade et al., A&A 594, A19 (2016), arXiv:1502.01594 [astro-ph.CO] .
- Pshirkov et al. [2016] M. S. Pshirkov, P. G. Tinyakov, and F. R. Urban, Physical review letters 116, 191302 (2016).
- Galanti and Roncadelli [2018b] G. Galanti and M. Roncadelli, Phys. Rev. D 98, 043018 (2018b).
- Belikov et al. [2011] A. V. Belikov, L. Goodenough, and D. Hooper, Phys. Rev. D 83, 063005 (2011).
- Franceschini et al. [2008] A. Franceschini, G. Rodighiero, and M. Vaccari, A&A 487, 837 (2008), arXiv:0805.1841 [astro-ph] .
- Kneiske and Dole [2010] T. M. Kneiske and H. Dole, A&A 515, A19 (2010), arXiv:1001.2132 [astro-ph.CO] .
- Domínguez et al. [2011a] A. Domínguez et al., Monthly Notices of the Royal Astronomical Society 410, 2556 (2011a), https://academic.oup.com/mnras/article-pdf/410/4/2556/6295256/mnras0410-2556.pdf .
- Gilmore et al. [2012] R. C. Gilmore, R. S. Somerville, J. R. Primack, and A. Domínguez, Monthly Notices of the Royal Astronomical Society 422, 3189 (2012), https://academic.oup.com/mnras/article-pdf/422/4/3189/18601416/mnras0422-3189.pdf .
- Inoue et al. [2013] Y. Inoue, S. Inoue, M. A. R. Kobayashi, R. Makiya, Y. Niino, and T. Totani, The Astrophysical Journal 768, 197 (2013).
- Franceschini and Rodighiero [2017] A. Franceschini and G. Rodighiero, A&A 603, A34 (2017), arXiv:1705.10256 [astro-ph.HE] .
- Saldana-Lopez et al. [2021] A. Saldana-Lopez, A. Domínguez, P. G. Pérez-González, J. Finke, M. Ajello, J. R. Primack, V. S. Paliya, and A. Desai, Monthly Notices of the Royal Astronomical Society 507, 5144 (2021), https://academic.oup.com/mnras/article-pdf/507/4/5144/40391540/stab2393.pdf .
- Domínguez et al. [2011b] A. Domínguez, M. Sánchez-Conde, and F. Prada, Journal of Cosmology and Astroparticle Physics 2011 (11), 020.
- Jansson and Farrar [2012a] R. Jansson and G. R. Farrar, The Astrophysical Journal 757, 14 (2012a).
- Jansson and Farrar [2012b] R. Jansson and G. R. Farrar, The Astrophysical Journal Letters 761, L11 (2012b).
- Adam et al. [2016] R. Adam et al., A&A 596, A103 (2016), arXiv:1601.00546 [astro-ph.GA] .
- Meyer et al. [2021] M. Meyer, J. Davies, and J. Kuhlmann, PoS ICRC2021, 557 (2021).
- Aleksić et al. [2016] J. Aleksić et al., Astroparticle Physics 72, 76 (2016), arXiv:1409.5594 [astro-ph.IM] .
- Ansoldi et al. [2018] S. Ansoldi et al., The Astrophysical Journal Letters 863, L10 (2018).
- Acciari et al. [2022] V. A. Acciari et al., The Astrophysical Journal 927, 197 (2022).
- Wood et al. [2017] M. Wood, R. Caputo, E. Charles, M. Di Mauro, J. Magill, J. S. Perkins, and Fermi-LAT Collaboration, in 35th International Cosmic Ray Conference (ICRC2017), International Cosmic Ray Conference, Vol. 301 (2017) p. 824, arXiv:1707.09551 [astro-ph.IM] .
- Banik and Bhadra [2019] P. Banik and A. Bhadra, Phys. Rev. D 99, 103006 (2019).
- Kelner and Aharonian [2008] S. R. Kelner and F. A. Aharonian, Phys. Rev. D 78, 034013 (2008).
- Böttcher et al. [2013] M. Böttcher, A. Reimer, K. Sweeney, and A. Prakash, ApJ 768, 54 (2013), arXiv:1304.0605 [astro-ph.HE] .
- Aharonian et al. [2008] F. A. Aharonian, D. Khangulyan, and L. Costamante, Monthly Notices of the Royal Astronomical Society 387, 1206 (2008), https://academic.oup.com/mnras/article-pdf/387/3/1206/3407041/mnras0387-1206.pdf .
- Vernetto and for the LHAASO Collaboration [2016] S. Vernetto and for the LHAASO Collaboration, Journal of Physics: Conference Series 718, 052043 (2016).
- Padovani et al. [2019] P. Padovani, F. Oikonomou, M. Petropoulou, P. Giommi, and E. Resconi, Monthly Notices of the Royal Astronomical Society: Letters 484, L104 (2019), https://academic.oup.com/mnrasl/article-pdf/484/1/L104/27711472/slz011.pdf .
- Zeng et al. [2013] H. Zeng, D. Yan, and L. Zhang, Monthly Notices of the Royal Astronomical Society 431, 997 (2013), https://academic.oup.com/mnras/article-pdf/431/1/997/18243934/stt223.pdf .
- Mauro et al. [2014] M. D. Mauro, F. Donato, G. Lamanna, D. A. Sanchez, and P. D. Serpico, The Astrophysical Journal 786, 129 (2014).
- Zhao et al. [2022] S. Zhao, R. Zhang, Y. Zhang, Q. Yuan, and LHAASO Collaboration, in 37th International Cosmic Ray Conference (2022) p. 859.
- Amenomori et al. [2021] M. Amenomori et al. (Tibet Collaboration), Phys. Rev. Lett. 126, 141101 (2021).
- Cherenkov Telescope Array Consortium et al. [2019] Cherenkov Telescope Array Consortium, B. S. Acharya, I. Agudo, I. Al Samarai, R. Alfaro, J. Alfaro, C. Alispach, R. Alves Batista, J. P. Amans, E. Amato, and et al., Science with the Cherenkov Telescope Array (2019).